以下参数的理解对PLL电路的学习和设计很重要。

- loop bandwidth

- vco gain

- damping factor

- natural frequency

- Q-factor

- closed-loop transfer function

- open-loop transfer function or gain

- loop forward gain

- radian frequency at which maximum phase margin located

- phase margin

loop bandwidth

    是表针PLL的open-loop gain 的unity-gain bandwidth。TI-snap003.pdf mentioned that loop bandwidth is the frequency at which the magnitude of loop gain or open-loop transfer function is equal to 1 or 0dB.(it was also confirmed in Banerjee, Dean. PLL performance, simulation and design. Dog Ear Publishing, 2006.) 

Wider loop bandwidth makes the PLL having a faster lock time but worse spurs. Choosing the loop bandwidth equal to the frequency where PLL noise and VCO noise are equal optimize the integrated phase noise.

PLL bandwidth

    定义为PLL的closed-loop transfer function的magnitude 等于-3dB时的频率. Matlab中可以用函数bandwidth直接得到f3dB。

(In many cases the bandwidth of a PLL is selected based on the minimum phase noise of the PLL output. This is determined by the trade-off between the phase noise that is translated to the output from the input reference and the noise generated internally by the PLL. In input reference dejitter applications, the spectrum of the noise of the input reference is higher than the internal noise of the PLL. In this case the loop bandwidth is set low so the input reference noise is rejected in the PLL loop filter. Conversely, if the noise of the reference is low relative to the PLL internal noise, then the loop gain of the PLL is increased to suppress internal noise, which also extends the PLL bandwidth. )

    PLL bandwidth is equal to loop bandwidth only when the feedback factor is equal to 1. When the feedback factor gets larger, the 3dB frequency becomes higher than loop bandwidth

VCO gain

    很好理解，就是VCO输入电压的变化所能引起VCO输出信号频率的变化大小。这个参数通常是无法改变的，当VCO设计好了之后。而在其设计之初，需要考虑好VCO的tuning range去定义VCO gain。

damping factor

   该参数跟pll step response的输出爬升有关。damping factor小，叫做under damping, 会有以下ripple/oscillaion现象; damping factor大, 叫做over damping, 无ripple, 可以看成一个single-pole system

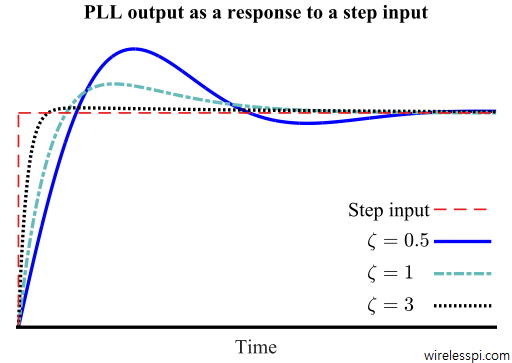
natural frequency

    该参数是表示pll 在没有锁定的时候的抖动情况。该参数大小跟锁定时间成反比。

需要注意的是，damping factor和natural frequency 都是对于second-order pll closed-loop transfer function的分母能简化成以下

如果想理解的更深一点，可以参考control system里面的second order system: https://ocw.mit.edu/courses/mathematics/18-03-differential-equations-spring-2010/readings/supp_notes/MIT18_03S10_chapter_13.pdf

type-II 2-order PLL, wn=sqrt(Kpd Kvco/NC1), damping factor = wn/2wz, wz = 1/RC1

当loop filter的阶数增加，就不能再用上述的式子计算natural frequency和damping factor了。准确的说，这两个参数只能用于type-II 2-order PLL.

Q-factor

    Q-factor是等于零点频率除以natural frequency, namely Q=wz/wn. 依然以type-II 2-order PLL为例，damping factor = 1/2Q。所以Q越小，damping factor越大。

说完damping factor, natural frequency, 对于type-II 2-order PLL, 它们跟BW有以下关系

当BW和damping factor 定了之后，natural frequency就可以计算出来。接着就可以去计算loop filter 和charge pump current等其他参数。

Dr. Dennis Fischette 在其PLL practise tutorial中讲到，natural frequency选择小于1/15 reference frequency 可以使PLL稳定性更好。

The above figure can help you understand the loop bandwidth, phase noise, damping factor and natrual frequency better, which was from PLL performance, simulation and design. 

closed-loop transfer function

    闭环转移函数，在我看来跟opamp negative feedback system很接近，或者说基本一样, (Acl=Aol/(1+Aol*beta)。把两者结合起来看，就会觉得很有意思。对于稳定性的判断，都是通过phase margin, poles, zeros 去分析的。需要注意的是，更高阶数的pll闭环转移函数计算loop bandwidth 越复杂。

open-loop transfer function

    开环转移函数，其实就是loop gain = forward gain * feedback factor. 在check phase margin/gain margin时，就需要得到开环转移函数。要理解这个，可以参考下图，它是type-II 3-order PLL的loop gain magnitude response. 此时，你不要跟opamp中的open loop gain混淆了，因为opamp中的open loop gain只是pll中的forward loop gain. 

    下图可以很好的看到，零点wz, natural frequency，unity-gain frequency(crossover frequency), pole frequency 

wz = 1/R1C1, wp = wzC2/(C1+C2)

forward loop gain

    该参数就是Kpd*Kvco/s*F(s)

radian frequency 和phase margin

    这两个参数要和在一起。radian frequency是对phase 求导，得到phase margin的最大值处的frequency。

对于type-II 3-order PLL， phase margin = tan-1(w0db/wz)-tan-1(w0db/wp3) 

为什么是wp3?因为前面两个极点都在origin。

以上是phase margin的公式，而要想使其最大，radian frequency =wz*sqrt(C1/C2+1)

需要注意的是，radian frequency是unity-gain frequency。但是不是所有的unity-gain frequency都是radian frequency.